
Finally, we propose a routingĪlgorithm that uses geographic routing in the greedy phase and virtual coordinates with backtracking to overcome voids andĪchieve high connectivity in the greedy phase with higher overall path quality and more resilience to localization errors. It also compares several existing routing protocols based on Virtual Coordinate systems. In addition, it is possible for nodes with the sameĬoordinates to arise at different points in the network in the presence of voids. Where packets reach nodes with no viable next hop in the forwarding set. However, we show that it is vulnerable to different forms of the void problem

That is more resilient to localization errors. Virtual coordinate systems which overlay a coordinate system on the nodes offer an alternative Geographical routing protocols have several desirable features for use in ad hoc and sensor networks but are susceptible to In this case the presented approach reaches optimal or best known solutions for hypercubes up to 131072 nodes and We have also modified our implementation to handle theoretically challenging large-scale classes of hypercubes and Hamming For smaller instances, GA solutions are compared with CPLEX results. GA relatively quickly obtains approximate solutions. Testing on instances with up to 1534 nodes shows that NP-hard problems: pseudo boolean, crew scheduling and graph coloring. For that reason, we present the results of the computational experience on several sets of test instances for other Metric dimension problem up to now has been considered only theoretically, standard test instances for this problem do notĮxist. The overall performance of the GA implementation is improved by a caching technique. The feasibility is enforcedīy repairing the individuals. (GA) that uses the binary encoding and the standard genetic operators adapted to the problem. And and we have that This is equal to matrix with four sub maitresse ease block matrix, zero block matrix.In this paper we consider the NP-hard problem of determining the metric dimension of graphs. So these are going to be different, said who called the 11 you want to all the way out to be one end 21 B 22 Onley out to be to end and then the M one b m. So it may not be that this is a symmetric matrix, and so we can consider that thes bottom and buy in entries may not be the transpose of the top end by em entries. And since we're not just considering simple graphs, we could also consider directed Gratz as well. We'll have some entries so called a 11 a 12 and still on all the way down to a one m 8 to 1 a two to a problem down to A to M and a n one they end to all the way down to a to No A and I m. So instead of just considering simple by partake graphs, suppose there would be multiple edges allowed, In which case we're not going to say ones. And we have, of course, that the way by partied graph works Is that in edge you the to virgin Cesaire adjacent if and only if one vertex lies and view one and another Vertex lies in the to so it follows that the first end by and block is going to be a block zeros Now it also follows that the and by M block in the bottom right corner is also going to be all zero and we have in the top, right? The end by M block is going to be all ones and in fact, weaken Generalize this. And then next we have you want you to all the way down to um And then, of course, across the top, same arrangement, the one the two up to the end. And so putting very sees from V one first, this is going to be the one to all the way down to the end.
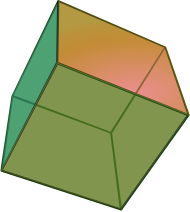
#Q4 HYPERCUBE PLUS#
Have form like so, first of all, because there are Enver Toussie's in V one and inverted Season two, and they're just joints total number of diseases n plus m This is going to be in n plus m by N plus and matrix.
The Vergis is so that toward various sees V one come first and then, and courtesies from B to then the adjacency matrix is going toe.

And M must be greater than a reporter one. So it follows that and must be greater than or equal to one. He's going had Burgess ease you want you to up through, um, and, of course, also these air known empty subsets. B one B two up through the end and feet, too. Be to such that he would envy, too, are just joint, and their union is he So will say V one has courtesies. The number of urgencies is greater than recorded, too, and it follows that you confined to subsets V one. Matrix has the form zero A zero for the four entries are rectangular blocks. We were asked to show the Vergis ease of a bay partite graph with two or more Vergis ease can be ordered so that it's adjacency.


 0 kommentar(er)
0 kommentar(er)
